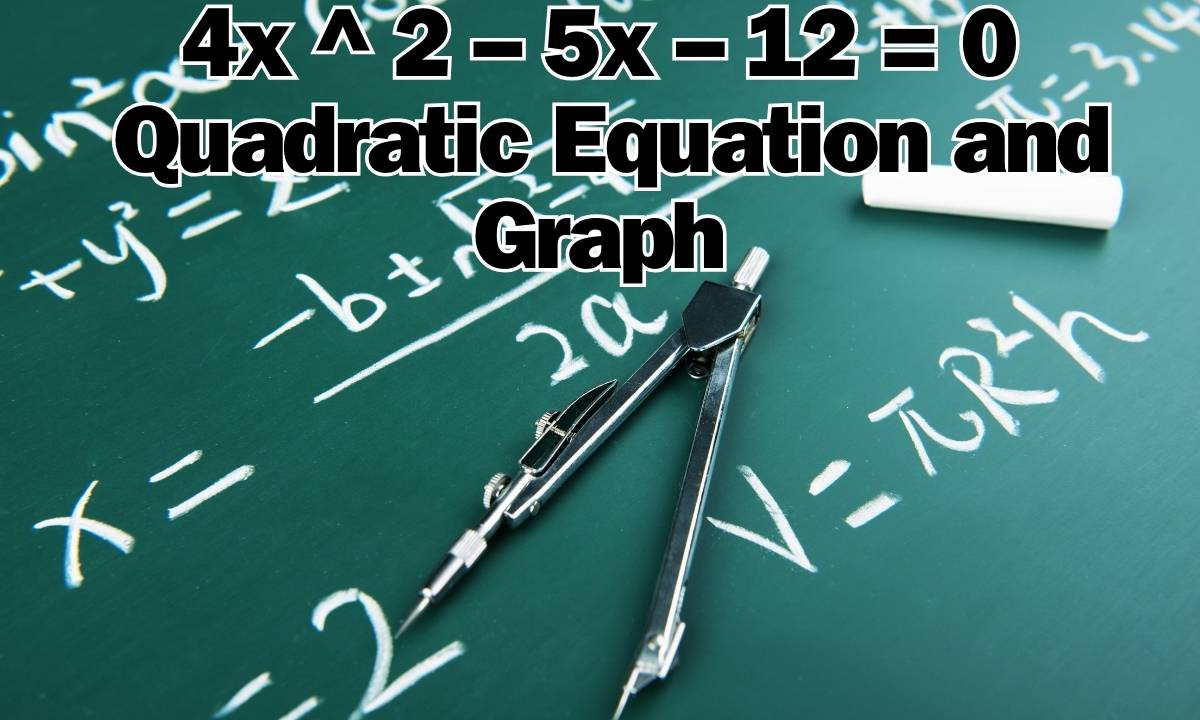
We will investigate and resolve the fascinating quadratic equation 4x ^ 2 – 5x – 12 = 0. Now a days, the quadratic equation plays a significant role in the math’s curriculum of all learning institutions, including colleges and universities.
Although many people would believe that quadratic has no real-time applications, this is not true because everything has important applications in a variety of other educational domains that are more based on research and development.
The Quadratic Equation 4x ^ 2 – 5x – 12 = 0
The quadratic equation is one of the most interesting concepts in algebra. Without knowing that, one may lose out on a significance component of learning about a crucial area of maths. This algebraic category is regarded as one of the essential ideas. We will examine quadratic equations in more detail in our post today, and we have included a thorough solution to 4x ^ 2 – 5x – 12 = 0.
The Quadratic Equation Concept
Any polynomial equation in the 2nd degree, which is similar to having at most one squared thing, could be classified as a quadratic equation. The quadratic equation can be expressed in the below general form: ax2 + bx + c = 0
Where x stands for the variable and a, b, and c are constants. Through this post, we will solve an equation and set it aside.
Equation:- 4x ^ 2 – 5x – 12 = 0
With the use of quadratic equation formulae, 4x ^ 2 – 5x – 12 = 0 equation is simply solved. This equation can be solved in two different ways: directly and via the Sridhar Acharya approach. Still, it is generally preferable to use the direct approach given this equation.
Answer of 4x ^ 2 – 5x – 12 = 0
There are two values of x that you will receive if you choose the direct way. Additionally, you’ll receive two values for x. This is a step-by-step learning that will help you answer this equation with simplicity. To learn more, continue reading:
The equation should be rewritten as a first step. Simply transcribe the equation into your notepad from your book or question paper.
Next, put your equation in writing using this format:
- 4x2-(2+3)x-12=0
After completing this, divide the equation’s center section and write it as follows:
- 4x2-2x-3x-12=0
Next, after combining the two parts, write your equation as follows:
- 2x(2x-1)-3(x-4) = 0
4x ^ 2 – 5x – 12 = 0 Solution through Sridhar Acharya
However, you’ll discover that this equation was invalid because the values you obtain after equating thus far are not acceptable.
Having stated that, there is an alternative approach to this sum: applying the Sridhar Acharya method. You will need to apply a formula in that manner, which is x = (-b ± √(b^2 – 4ac)) / 2a.
Here, we must enter the required values from the equation for x, a, and c and then substitute them into this equation. You will eventually determine the required values if you continue to calculate.
- (-b ± √(b2 – 4ac))/2a = x
- Let put a = 4,
- b = 5,
- and c = 12;
Now let’s the value of a,b,c in Sridhar Acharya formula we get => x = (-5 ± √(52 – 4x4x12))/2×4
Once the equation above is solved, we obtain x= √217 + 5/8 or x= − √217 + 5/8.
Moreover, we obtain the Axis of Symmetry (dashed) {x}={ 0.62} after computing the root value.
- At {x,y} = {0.62,-13.56}, vertex
- x-Intercepts at the Roots:
- {x,y} = {-1.22, 0.00} At Root 1.
- {x,y} = { 2.47, 0.00} At Root 2.
Hence, you get x= -1.22 and x= 2.47 on solving the 4x2– 5x – 12 = 0 and determine the values for x in this manner.
Graph of 4x2– 5x – 12 = 0:-

Quadratic Equation Applications
Although quadratic equations do not seem like they are normally useful in today’s society, they are actually more important for a number of add-on things. The below are the list of situations in which quadratic equations are very important:
One of the disciplines that uses these equations to carry out significant computations is physics. Projectile motion equations and other key physics concepts are calculated using quadratic equations.
Quadratic equations are frequently used in engineering to solve equations. These formulas may relate to electrical circuits, signal processing, or structural analysis.
Quadratic equations are used in the study of finance, despite the fact that this may seem unusual to hear, particularly when modeling intricate financial systems and calculating tax investment returns.
Also, Read About:-